Q1.
ABC is right triangle right angled at B, such that AB = c, AC = b, BC = a. find the radius of its incircle.
(a) (b + c)/2 (b) a - b (c) a + b (d) (c + a - b)/2
Solution.

r =
AB + BC - AC/2
r =
c + a - b/2
Solution
Q2.
∠BAC = 90o , and segment AD ⊥ BC Then AD2 =
(a) BD x DC (b) BD2
(c) DC2 (d) BC
Solution.
(a) BD x DC

Solution
Q3.
If triangle of ABC is similar to the triangle of DEF such that BC = 3 cm, EF = 4 cm and area of triangle of ABC = 362 . Determine
the area of triangle of DEF = ____
(a) 86 cm2 (b) 64 cm2 (c) 60 cm2
(d) 74 cm
Solution.
Area of triangle ABC/Area of triangle DEF
=
(BC)2/(EF)2
36/x
=
9/16
x = 64 cm
2
Solution
Q4.
Find the value of θ
√3 tan2 θ - 3 = 0
(a) 60o (b) 50o
(c) 30o (d) 40o
Solution.
tan2 θ = 3/√3 = √3 = tan60o
2 θ = 60o
θ = 30o
Solution
Q5.
If A,B,C are the interior angled of a triangle ABC , sin(B + C)/2
(a) cot(A/2) (b) sin(A/2) (c) cos (A/2) (d) 4
Solution.
(c) cos (A/2)
Solution
Q6.
cos A + coa2 A = 1, then sin2A + sin4 A = 1
(a) 1 (b) 0 (c) -1 (d) 2
Solution.
(a) 1
Solution
Q7.
The area of a sector whose perimeter is four times its radius r units is.
(a) r4 sq.unit (b) r2 sq.unit
(c) r sq.unit (d) r3 sq.unit
Solution.
Area of sector = 1/2 rl
1/2(r)(2r) = r2 sq.unit
Solution
Q8.
Find the area of triangle formed by(a,b+c)(b,c+a)(c,a+b)
(a) 0 (b) 1 (c) 2 (d) 3
Solution.
(a) 0
Solution
Q9.
Find the area of the shaded portion
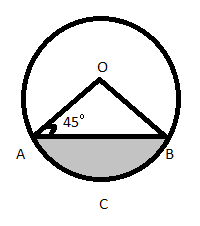
(a) 2π - 4 (b) r2/4(π - 2)
(c) r/2(π - 2) (d) (π - 2)
Solution.
Area of ACB = Area of sector OACB - Area of triangle OAB
90/360 π r2 - 1/2 r2
= r2/4(π - 2)
Solution
Q10.
The perimeter of a sector of a circle of radius π is (5 π)/2 . find the area of the sector .
(a)
2 π2 (b) (1/2) π2 (c) π2 (d) (1/4) π2
Solution.
2r + arc length = (5π)/2
2π + arc length = (5π)/2
arc length = (5π)/2 - 2π
Area of the sector = (1/2) rl = (1/2)π x (π/2)
= (1/4) π2
Solution




